Finding the number of ways to climb the stairs - Part 1

Problem

You are climbing a stair case. Each time you can either make 1 step or 2 steps. The staircase has n steps. In how many distinct ways can you climb the staircase ?

For example, If there are 3 stairs, there are three possible ways to climb them.

{1,1,1}, {1, 2}, {2, 1}

Your algorithm should print out the total number of ways to climb the stairs. (Optional: should also print out the different ways as above)

I will first start with the brute force approach, which does not give us ideal time complexity.
I will cover the better algorithm in Part 2. So stay tuned.

Given the two choices, 1 step or 2 steps, you can draws the Binary trees as below.

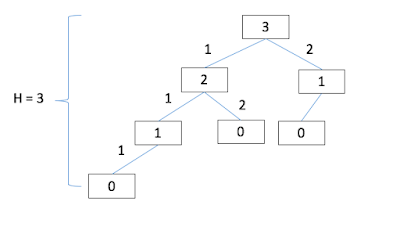Binary Tree to find ways to climb stairs

What we need to do is to perform DFS on the binary tree to find the case where node is zero and record the path.

Running time of DFS is O(M), where M = 2^N, where N is the number of stairs.
Therefore, running time of the algorithm is O( 2^ N).
Here is the complete code running in O( 2^N).

	#include<list>
	#include<string>
	#include<iostream>
	using namespace std;
	void permutate(string cur, int left, list<string>&result)
	{
	if (left <= 0)
	{
	if (left == 0)
	result.push_back(cur);
	return;
	}
	left -=1;
	cur.push_back('1');
	permutate(cur, left, result);
	left+=1;
	cur.pop_back();
	left -=2;
	cur.push_back('2');
	permutate(cur, left, result);
	left+=2;
	cur.pop_back();
	}
	void list_ways_to_climb_stairs(int num_stairs)
	{
	list<string> result;
	list<string>::iterator iter;
	permutate("", num_stairs, result);
	for(iter = result.begin(); iter != result.end(); iter++)
	cout<<*iter<<endl;
	}
	int main()
	{
	list_ways_to_climb_stairs(5);
	return 1;
	}

view raw find_ways_to_climb_stairs.cpp hosted with ❤ by GitHub

Above algorithm give us what we want but its running time O(2^N) is not ideal.
Can we do better ? I think so, let find a better algorithm in Part 2.

Practice statistics
23 mins: to write up the code
3 mins: to fix the typos through compilation.